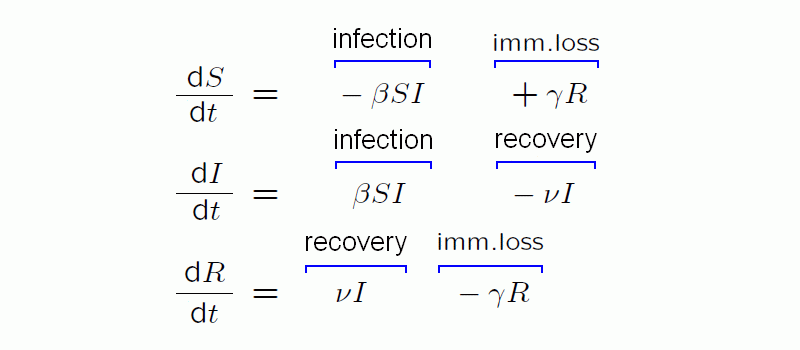感染症疫学に関する数理モデルのひとつの標準型
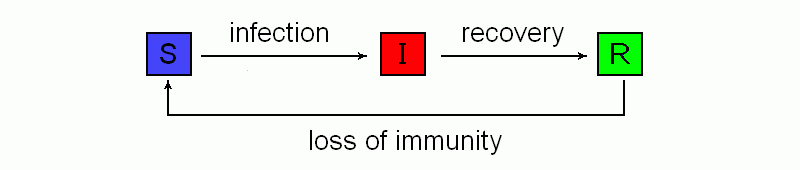
対象とする個体群の各個体は3つの状態
S:susceptible 感受性個体で感染する可能性のある状態
I:infected 感染して感染性を有する状態
R:recovered(immune) 感染後に回復して免疫を獲得した状態
の内のどれか1つの状態をとる.これら3つの状態は次のように変わっていく:
最も簡単なのは,時刻 t に状態 “susceptible”,“infected”,“recovered”にある個体数
をそれぞれ S(t),I(t),R(t) として,連立微分方程式
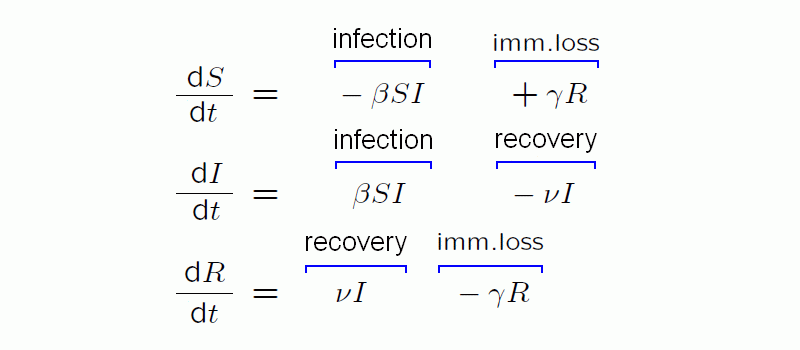
による決定論的モデルである.
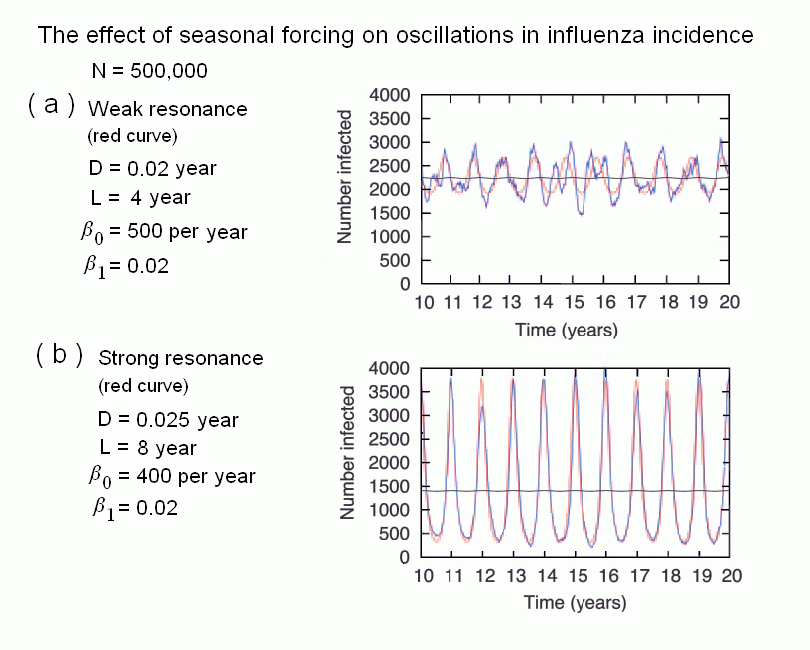
Dushoff et al.による少し拡張した適用例
Dynamical resonance can accout for seasonality of influenza epidemics
J.Dushoff, J.B.Plotkin, S.A.Levin and D.J.D.Earn
Proc Natl Acad USA November 30,2004 vol.101 no.48 16915-16916
http://www.pubmedcentral.nih.gov/articlerender.fcgi?artid=534740
Abstract Influenza incidence exhibits strong seasonal fluctuations in temperate regions throughout
the world, concentrating the mortality and morbidity burden of the disease into a few months each year. The cause of influenza's
seasonality has remained elusive. Here we show that the large oscillations in incidence may be caused by undetectably
small seasonal changes in the influenza transmmision rate that are amplified by dynamical resonance.